Remember that time in algebra class when your teacher introduced inequalities with a grin, saying, “Now things are getting interesting!”? Those multi-step inequalities, with their variables tucked away on both sides of the equation and a hefty dose of operations, can feel intimidating. But fear not! This 5-3 study guide and intervention will equip you with the tools and confidence to tackle them head-on. It’s time to unlock the power of inequalities and see the world of math in a whole new light.

Image: www.pdfprof.com
Think back to a time when you wanted to achieve a goal, whether it was saving money for a new bike or getting a certain grade in a class. To reach those milestones, you needed to understand the constraints, or boundaries, that existed. Inequalities are like those invisible boundaries in the realm of math. They help us define the range of possible solutions to a problem. So, whether you’re solving a real-life budget equation or a complex math problem, understanding inequalities becomes a valuable skill.
Delving Deeper into the World of Inequalities
The Foundation: What are Inequalities?
Inequalities are mathematical expressions that compare two values using symbols like:
- < (less than)
- > (greater than)
- ≤ (less than or equal to)
- ≥ (greater than or equal to)
For example, “x < 5” means that the value of x is any number smaller than 5.
The Key to Solving Multi-Step Inequalities
The core principle behind solving multi-step inequalities mirrors the process of solving equations. However, there’s one crucial twist – flipping the inequality sign! Confused? Here’s the breakdown:
- Multiplying or Dividing by a Negative Number: Imagine you have a statement saying “5 is greater than 3.” Now, multiply both sides by -1. Suddenly, the statement becomes “-5 is less than -3.” This is where the magic of flipping the inequality sign comes into play. Whenever you multiply or divide both sides of an inequality by a negative number, you must flip the inequality sign to maintain the accuracy of the solution.
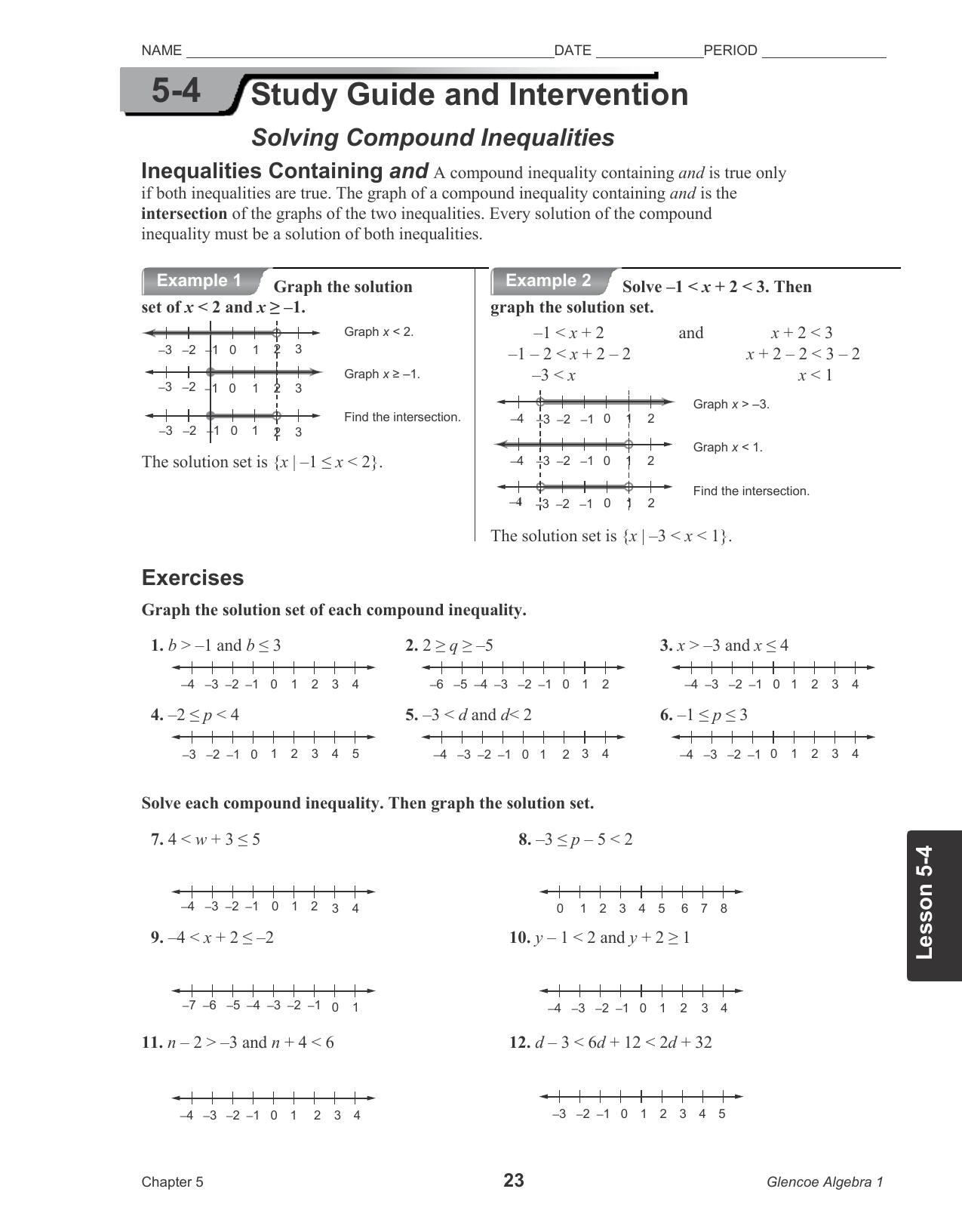
Image: studyposter.blogspot.com
The Steps of Success: A 5-3 Approach
Think of the “5-3” approach as your blueprint for mastering multi-step inequalities.
Step 1: Simplify Each Side This involves combining like terms, distributing, and performing any necessary operations to simplify the left and right sides of the inequality.
Step 2: Isolate the Variable This is where the action takes place. To get the variable by itself on one side of the inequality, you’ll use inverse operations (addition/subtraction, multiplication/division) just like you would with equations.
Step 3: Combine Like Terms Gather all the variable terms on one side and all the constant terms on the other.
Step 4: Isolate the Variable Term Use division or multiplication to isolate the variable. Remember to flip the inequality sign if you multiply or divide by a negative number!
Step 5: Determine the Solution The final step is to express your solution in a clear and concise way. For example, if the solution is “x < 7,” you might write:
- Solution: The solution set is all numbers less than 7.
- Graphically: You could represent the solution on a number line with an open circle at 7 and an arrow extending to the left, indicating all numbers less than 7.
- Interval Notation: You would express the solution set as (-∞, 7).
Unlocking the Power of Examples
Let’s bring these concepts to life with an example:
Solve the inequality: 3x – 5 > 2x + 1
- Simplify Each Side: The inequality is already simplified.
- Isolate the Variable: Subtract 2x from both sides:
- 3x – 5 – 2x > 2x + 1 – 2x
- x – 5 > 1
- Combine Like Terms: The variable terms are combined.
- Isolate the Variable Term: Add 5 to both sides:
- x – 5 + 5 > 1 + 5
- x > 6
- Determine the Solution: The solution set is all numbers greater than 6.
Mastering Multi-Step Inequalities: Beyond the Basics
When tackling multi-step inequalities, you might encounter some additional challenges. Here are some helpful tricks to keep in mind:
- Fractions: If you encounter fractions, you can get rid of them by multiplying both sides of the inequality by the least common multiple (LCM) of the denominators.
- Grouping Symbols: Just like in solving equations, work from the inside out when dealing with parentheses or brackets. Distribute if needed, and remember to apply the properties of inequalities when dealing with negative values.
- Absolute Value Inequalities: Absolute value inequalities involve expressions like |x – 2| > 5. These inequalities require a bit more attention.
- You’ll need to consider two cases: one where the expression inside the absolute value is positive and another where it’s negative.
- Solve each case separately, and then combine the solutions to get the final answer.
Expert Insights and Actionable Tips
Just like a seasoned chef carefully blends ingredients to create a delicious dish, a successful mathematician combines foundational knowledge with strategic thinking. Here are some tips from math experts:
- Visual Representation: Don’t underestimate the power of visualizing solutions. Use number lines or graphs to understand the range of possible values that satisfy an inequality.
- Practice Makes Perfect: Just like you wouldn’t become a master chef by only reading cookbooks, mastering inequalities requires practice. Work through various examples to build confidence and develop your intuition.
5-3 Study Guide And Intervention Solving Multi-Step Inequalities
The Final Word: Unleash Your Mathematical Power
By conquering the world of multi-step inequalities, you’re not just mastering a mathematical concept – you’re honing your analytical skills, your problem-solving prowess, and your ability to think critically. Remember, understanding inequalities allows you to analyze constraints, interpret data, and model real-world situations with greater precision. So, take your newfound knowledge, and apply it to your studies, your career, or even to everyday decision-making. The power of math is in your hands, waiting to be unleashed.






