Ever wondered how a rollercoaster designer knows how steep to make each drop? Or how a carpenter can build a perfectly slanted roof? The answer lies in the fascinating world of slopes! Understanding slopes is essential in various fields, from engineering to architecture. This guide will take you on a journey to master the basics of slope calculations, delve into 3-3 skills practice problems, and unlock the secrets behind this fundamental concept.

Image: materialoster.z21.web.core.windows.net
This guide is tailored for students who are navigating the world of linear functions and are ready to conquer the concept of slopes. It will help you develop problem-solving skills, understand the practical applications of slopes, and build a strong foundation for future mathematical studies. So, let’s dive right in and explore the world of slopes together!
Understanding the Basics: What is Slope?
Imagine a straight line drawn on a graph. This line represents a relationship between two variables, usually displayed on the x-axis and y-axis. The slope of this line is like its “steepness” – how much it rises or falls for every unit it moves horizontally. It’s a measure of how quickly the line changes direction.
Mathematically, slope is calculated as the ratio of the vertical change (rise) to the horizontal change (run) between any two points on the line. The formula for calculating slope is:
Slope = (Change in y) / (Change in x) = (y2 – y1) / (x2 – x1)
The slope can be positive, negative, zero, or undefined. A positive slope indicates an upward incline from left to right, while a negative slope indicates a downward incline. A slope of zero represents a horizontal line, and an undefined slope signifies a vertical line.
3-3 Skills Practice: Sharpening Your Slope Skills
Now, let’s put our knowledge into practice! The 3-3 skills practice approach focuses on mastering the concept of slope through a series of three distinct steps:
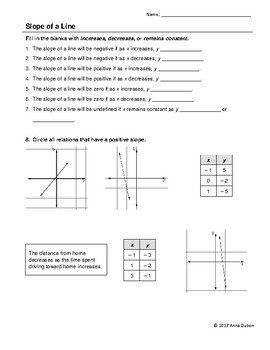
Image: www.teacherspayteachers.com
Step 1: Conceptual Understanding
To begin, let’s solidify our understanding of slope. Here are some key concepts to consider:
- Slope as a ratio: Remember the formula – slope is the ratio of “rise” over “run.” Visualizing this helps to grasp the concept intuitively.
- Positive, negative, zero, and undefined slopes: Understand the implications of each type of slope and how it reflects the direction of the line.
- Slope-intercept form: The equation y = mx + b, where ‘m’ represents the slope and ‘b’ represents the y-intercept, provides a powerful tool for analyzing and interpreting linear functions.
Step 2: Practical Application
Let’s move on to applying our understanding of slope in real-world scenarios. Here are some examples:
- Finding the slope of a line: Given two points on a line, calculate the slope using the formula.
- Determining the slope from an equation: Identify the slope (m) from the slope-intercept form of the equation (y = mx + b).
- Interpreting slope in context: Analyze a situation where slope plays a role, such as the speed of a moving object or the rate of growth in a population.
Step 3: Problem-Solving Practice
The final step is tackling various types of problems related to slopes. Here are some examples:
- Finding the equation of a line given a point and a slope: Use the point-slope form of a linear equation (y – y1 = m(x – x1)).
- Determining the slope of a line from a graph: Identify two points on the line and calculate the slope.
- Solving word problems involving slopes: Apply the concept of slope to solve real-world scenarios, such as calculating the rate of change in distance or finding the angle of a ramp.
Examples Galore: Deepening Your Understanding
Let’s delve into some specific examples to illustrate the concepts we’ve discussed:
Example 1: Finding the slope of a line
Suppose we have two points on a line: (2, 3) and (5, 7). Using the slope formula, we get:
Slope = (7 – 3) / (5 – 2) = 4/3
This means that for every 3 units the line moves horizontally, it rises by 4 units.
Example 2: Determining the slope from an equation
Take the equation y = -2x + 5. The slope-intercept form tells us that the slope (m) is -2. This means the line has a downward incline, decreasing 2 units vertically for every unit it moves horizontally.
Example 3: Solving a word problem
Imagine a car traveling at a constant speed. Let the distance traveled be represented by ‘y’ and the time taken be ‘x’. If the car travels 100 kilometers in 2 hours, what is the car’s speed?
Speed is calculated as distance over time, which translates to the slope in this scenario. So, the speed is 100 kilometers / 2 hours = 50 kilometers per hour. This is how the concept of slope applies to real-world scenarios.
Beyond the Basics: Advanced Topics in Slopes
As you progress in your mathematical journey, the concept of slope expands into more complex applications. Here are some advanced topics to explore:
- Parallel and perpendicular lines: Understanding the relationship between the slopes of parallel and perpendicular lines is crucial for solving geometric problems.
- Lines of best fit: Using regression analysis and linear functions, we can find the ‘line of best fit’ to represent trends in data sets.
- Higher-dimensional spaces: Slope extends beyond two-dimensional graphs and finds application in higher-dimensional spaces, essential for visualizing and understanding complex mathematical relationships.
3-3 Skills Practice Slopes Of Lines
Conclusion: Mastering Slopes for a World of Opportunities
This guide has provided a comprehensive overview of slopes, covering basic definitions, practical applications, and problem-solving strategies. By mastering the concepts of slope, you open doors to various disciplines, from engineering and architecture to economics and data analysis. The skills you acquire will prove invaluable in understanding and interpreting patterns, making predictions, and solving real-world challenges. Remember, the journey of mastering slopes is a continuous one. Don’t hesitate to explore further resources, experiment with different problems, and seek guidance when needed. As you delve deeper, you’ll discover the profound impact of slopes in shaping our understanding of the world around us.






